Koyo Technical Information
3. Bearing service life and selection
a. Basic dynamic load rating
1) Bearing service life
When bearings rotate under loading, material flakes from the surfaces of inner and outer rings or rolling elements by fatigue arising from repeated contact stress. This phenomenon is called flaking. The total number of bearing rotations until flaking occurs is regarded as the bearing “(fatigue) service life”. “(Fatigue) service life” differs greatly depending upon bearing structures, dimensions, materials, and processing methods. Since this phenomenon results from fatigue distribution in bearing materials themselves, differences in bearing service life should be statistically considered. When a group of identical bearings is rotated under the same conditions, the total number of revolutions until 90% of the bearings are left without flaking (i.e. a service life of 90% reliability) is defined as the basic rating life. In operation at a constant speed, the basic rating life can be expressed in terms of time.
In actual operation, a bearing fails because of not only fatigue, but other factors as well, such as wear, seizure, creeping, fretting, brinelling, cracking etc (ref. Bearing failures and countermeasures). Selecting the proper mounting method and lubricant, as well as the bearing most suitable for the application can minimize these bearing failures.
2) Basic dynamic load rating
The basic dynamic load rating is either pure radial (for radial bearings) or central axial load (for thrust bearings) of constant magnitude in a constant direction, under which the basic rating life of 1 million revolutions can be obtained, when the inner ring rotates while the outer ring is stationary, or vice versa. The basic dynamic load rating, which represents the capacity of a bearing under rolling fatigue, is specified as the basic dynamic radial load rating (Cr) for radial bearings, and basic dynamic axial load rating (Ca) for thrust bearings. These load ratings are listed in the specification table.
3) Dynamic equivalent load
Bearings are used under various operating conditions; however, in most cases, bearings receive radial and axial load combined, while the load magnitude fluctuates during operation. The two are compared by replacing the load applied to the shaft center with one of a constant magnitude and in a specific direction, that yields the same bearing service life as under actual load and rotational speed. This theoretical load is reffered to as the dynamic equivalent load, can be obtained using the equivalent load equation.
- Deep groove ball bearings
Dynamic equivalent radial load
Pr=XFr+YFa
| f0Fa/C0r | e | Fa/Fr ≤ e | Fa/Fr >e | ||
|---|---|---|---|---|---|
| X | Y | X | Y | ||
| 0.172 | 0.19 | 1 | 0 | 0.56 | 2.30 |
| 0.345 | 0.22 | 1.99 | |||
| 0.689 | 0.26 | 1.71 | |||
| 1.03 | 0.28 | 1.55 | |||
| 1.38 | 0.30 | 1.45 | |||
| 2.07 | 0.34 | 1.31 | |||
| 3.45 | 0.38 | 1.15 | |||
| 5.17 | 0.42 | 1.04 | |||
| 6.89 | 0.44 | 1 | |||
Factor f0 is shown in the bearing dimension table.
Static equivalent radial load
P0r=0.6Fr+0.5Fa
when P0r<Fr ; P0r=Fr
- Angular contact ball bearings – Single-row angular contact ball bearings
Dynamic equivalent radial load
Pr=XFr+YFa
| Contact angle |
if0Fa/C0r | e | Single-row and tandem arrangement | Back-to-back and face-to-face arrangement | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Fa/Fr≤e | Fa/Fr>e | Fa/Fr≤e | Fa/Fr>e | |||||||
| X | Y | X | Y | X | Y | X | Y | |||
| α =15° | 0.178 0.357 0.7141.07 1.43 2.143.57 5.35 5.35 |
0.38 0.40 0.430.46 0.47 0.500.55 0.56 0.56 |
1 | 0 | 0.44 | 1.47 1.40 1.301.23 1.19 1.121.02 1.00 1.00 |
1 | 1.65 1.57 1.461.38 1.34 1.261.14 1.12 1.12 |
0.72 | 2.39 2.28 2.112.00 1.93 1.821.66 1.63 1.63 |
| α =30° | – | 0.8 | 1 | 0 | 0.39 | 0.76 | 1 | 0.78 | 0.63 | 1.24 |
| α =40° | – | 1.14 | 1 | 0 | 0.35 | 0.57 | 1 | 0.55 | 0.57 | 0.93 |
For i, use 2 for DB & DT arrangements and 1 for single & DT arrangement.
Factor f0 is shown in the bearing dimension table.
Static equivalent radial load
P0r=X0Fr+Y0Fa
In reference to single-row or tandem arrangement bearings,
when P0r<Fr; P0r=Fr
| Contact angle |
Single-row and tandem arrangementt | Back-to-back and face-to-face arrangement | ||
|---|---|---|---|---|
| X0 | Y0 | X0 | Y0 | |
| α =15° | 0.5 | 0.46 | 1 | 0.92 |
| α =30° | 0.5 | 0.33 | 1 | 0.66 |
| α =40° | 0.5 | 0.26 | 1 | 0.52 |
- Angular contact ball bearings – Double-row angular contact ball bearings
Dynamic equivalent radial load
Pr=XFr+YFa
| Contact angle |
e | Fa/Fr≤e | Fa/Fr>e | ||
| X | Y | X | Y | ||
| α =24° | 0.66 | 1 | 0.95 | 0.68 | 1.45 |
| α =32° | 0.86 | 1 | 0.73 | 0.62 | 1.17 |
Static equivalent radial load
P0r=X0Fr+Y0Fa
| Contact angle | X0 | Y0 |
| α =24° | 1 | 0.78 |
| α =32° | 1 | 0.63 |
- Self-aligning ball bearings
Dynamic equivalent radial load
Pr=XFr+YFa
| Fa/Fr≤e | Fa/Fr>e | ||
| X | Y | X | Y |
| 1 | Y1 | 0.65 | Y2 |
Refer to the bearing specification table for values of e, Y1, Y2.
Static equivalent radial load
P0r=Fr+Y0Fa
Refer to the bearing specification table for value of Y0.
- Cylindrical roller bearings
Dynamic equivalent radial load
Pr=Fr
Static equivalent radial load
P0r=Fr
- Tapered roller bearings – Single-row
Dynamic equivalent radial load
when Fa/Fr≤e ; Pr=Fr
when Fa/Fr>e ; Pr=0.4Fr+Y1Fa
Refer to the bearing specification table for the values of axial load factors Y1 and constant e.
Static equivalent radial load
P0r=0.5Fr+Y0Fa
when P0r<Fr ; P0r=Fr
Refer to the bearing specification table for the value of axial load factors Y0.
- Tapered roller bearings – Double-row / four-row
Dynamic equivalent radial load
when Fa/Fr≤e ; Pr=Fr+Y2Fa
when Fa/Fr>e ; Pr=0.67Fr+Y3Fa
Refer to the bearing specification table
for the values of axial load factors Y2, Y3 and constant e.
Static equivalent radial load
P0r=Fr+Y0Fa
Refer to the bearing specification table for the value of axial load factors Y0.
- Spherical roller bearings
Dynamic equivalent radial load
when Fa/Fr≤e ; Pr=Fr+Y1Fa
when Fa/Fr>e ; Pr=0.67Fr+Y2Fa
Refer to the bearing specification table
for the values of axial load factors Y1, Y2 and constant e.
Static equivalent radial load
P0r=Fr+Y0Fa
Refer to the bearing specification table for the values of axial load factors Y0 and constant e.
- Needle roller bearings
Dynamic equivalent radial load
Pr=Fr
Static equivalent radial load
P0r=Fr
- Thrust ball bearings
Dynamic equivalent axial load
Pa=Fa
Static equivalent axial load
P0a=Fa
- Spherical thrust roller bearings
Dynamic equivalent radial load
Pa=1.2Fr+Fa
Static equivalent axial load
P0a![]() 2.7Fr+Fa
2.7Fr+Fa
Note : Fr/Fa≤0.55
4) Basic rating life
The basic rating life in relation to the basic dynamic load rating and dynamic equivalent load can be expressed using equation (3-1). It is convenient to express the basic rating life in terms of time, using equation (3-2), when a bearing is used for operation at a constant speed; and, in terms of mileage (km), using equation (3-3), when a bearing is used in railway rolling stock or automobiles.
| (Total revolutions) | L10 | = (C/P)p ⋯ | (3-1) |
| (Time) | L10h | = 106(C/P)p/60n ⋯ | (3-2) |
| (Running distance) | L10s | = π DL10 ⋯ | (3-3) |
| where: | ||||
| L10 | : basic rating life | 106 (revolution) | ||
| L10h | : basic rating life | h | ||
| L10s | : basic rating life | km | ||
| P | : dynamic equivalent load | N | ||
| C | : basic dynamic load rating | N | ||
| n | : rotational speed | min-1 | ||
| p | : for ball bearings ⋯ | p=3 | ||
| : for roller bearings ⋯ | p=10/3 | |||
| D | : wheel or tire diameter | mm | ||
5) Correction of calculated service life
When the bearing is used under heat, adjust the service life by multiplying the basic dynamic load rating indicated in the Bearing Specification Tables by the temperature adjustment factor.
Values of Temperature Adjustment Factor
| Bearing temperature °C | 125 | 150 | 175 | 200 | 250 |
|---|---|---|---|---|---|
| Temperature adjustment factor | 1 | 1 | 0.95 | 0.90 | 0.75 |
For applications where more than one bearing is used, calculate the service life of the entire bearing system.
Equation for Calculation of Service Life of Entire System
π ![]()
| where: | ||
| i>L : | Rating life of the entire bearing system | |
| L1,L2,L3 ⋯: | Rating life of individual bearings | |
|
e :
|
Constant | e =10/9 ⋯ for ball bearings |
| e = 9/8 ⋯ for roller bearings | ||
| If both types of bearings are used, use an average. | ||
Also, adjust the rating life by using reliability factor a1, bearing characteristic factor a2, and operating condition factor a3:
1) Reliability factor a1
The following table describes reliability factor, a1, which is necessary to obtain the corrected rating life of reliability greater than 90%.
| Reliability, % | a1 |
|---|---|
| 90 | 1 |
| 95 | 0.62 |
| 96 | 0.53 |
| 97 | 0.44 |
| 98 | 0.33 |
| 99 | 0.21 |
2) Bearing characteristic factor a2
The bearing characteristic in relation to bearing life may differ according to bearing materials (steel types and their quality), and may be altered by production process, design, etc. In such cases, the bearing life calculation can be corrected using the bearing characteristic factor a2.
Koyo has employed vacuum-degassed bearing steel as our standard bearing materials. It has a significant effect on bearing life extension, which was verified through studies at our laboratory. The basic dynamic load rating of bearings made of vacuum-degassed bearing steel is specified in the bearing specification table, taking the bearing characteristic factor as a2=1. For bearings made of special materials to extend fatigue life, the bearing characteristic factor is treated as a2>1.
3) Operating condition factor a3
When bearings are used under operating conditions, which directly affect their service life, including improper lubrication, the service life calculation can be corrected by using a3.
Under normal lubrication, the calculation can be performed with a3=1; and, under favorable lubrication, with a3>1.
In the following cases, the operating condition factor is treated as a3<1:
- Operation using lubricant of low kinematic viscosity
For ball bearings … 13mm2/s or less
For roller bearings … 20mm2/s or less
- Operation at very slow rotation speed
Product of rolling element pitch diameter and rotational speed is 10000 (mm![]() m/min) or less.
m/min) or less.
- Contamination of lubricant is expected
- Greater misalignment of inner and outer rings is present
For calculation of the service life in due consideration of a2 and a3, use the Calculation Menu entitled Life Calculation.
b. Selection based on service life
The following is an example of how an appropriate bearing can be selected based on the required service life. To determine required service life in actual cases, refer to the selection menu.
1) Initial conditions
Bearing radial load (Fr), bearing axial load (Fa), rotational speed (n), and bearing bore diameter (d)
2) Selection of bearing type
Select a proper type of bearing from among those listed in the Example Bearing Arrangements.
- Arrangement in which fixed and free sides are distinguished
n : Rotation speed; Fr : Radial load; Fa : Axial load; Q : Aligning capability
| Bearing arrangement | n | Fr | Fa | Q | Application example | |
|---|---|---|---|---|---|---|
| Fixed side | Free side | |||||
 |
 |
High | Light | Light | Low | Medium size motors, air blowers |
 |
 |
High | Heavy | Light | Low | Traction motors for railway rolling stock |
 |
 |
High | Heavy | Heavy | Low | Speed reducers, lathe spindles |
 |
 |
High | Normal | Normal | Low | Motors |
 |
 |
Middle | Heavy | Normal | Middle | Paper manufacturing calender rollers |
 |
 |
High | Heavy | Light | Low | Speed reducers |
 |
 |
High | Light | Light | Low | Pumps |
 |
 |
High | Light | Normal | Low | Worm gear, speed reducers |
 |
 |
Middle | Heavy | Normal | High | Steel manufacturing table rollers |
- Arrangement in which fixed and free sides are not distinguished
n : Rotation speed; Fr : Radial load; Fa : Axial load; Q : Aligning capability
| Bearing arrangement | n | Fr | Fa | Q | Application example |
|---|---|---|---|---|---|
 |
High | Light | Light | Low | Small motors, small pumps |
 Back-to-back Back-to-back Face-to-face Face-to-face |
High | Light | Normal | Low | Machine tool spindles |
 Back-to-back Back-to-back Face-to-face Face-to-face |
High | Heavy | Heavy | Low | Speed reducers, automobile wheels |
 |
High | Normal | Normal | Low | Machine tool spindles |
 |
High | Heavy | Light | Low | Construction equipment |
- Application to vertical shafts
n : Rotation speed; Fr : Radial load; Fa : Axial load; Q : Aligning capability
| Bearing arrangement | n | Fr | Fa | Q | Application example |
|---|---|---|---|---|---|
 Fixed side Fixed side  Free side Free side |
High | Normal | Normal | Low | Vertical motors |
 Free side Free side Fixed side Fixed side |
Middle | Heavy | Heavy | High | Crane center shafts |
3) Determination of required service life
Determine the required service life of bearings, referring to Table 3-1.
Table 3-1 Recommended bearing service life
| Operating condition | Application | Recommended service life (h) |
|---|---|---|
| Short or intermittent operation | Household electric appliance, electric tools,agricultural equipment, heavy cargo hoisting equipment | 4,000 to 8,000 |
| Not extended duration, but stable operation required | Household air conditioner motors,construction equipment, conveyers, elevators | 8,000 to 12,000 |
| Intermittent but extended operation | Rolling mill roll necks, small motors,cranes | 8,000 to 12,000 |
| Motors used in factories, general gears | 12,000 to 20,000 | |
| Machine tools, shaker screens, crushers | 20,000 to 30,000 | |
| Compressors, pumps, gears for essential use | 40,000 to 60,000 | |
| Daily operation more than 8 hrs. or continuous extended operation | Escalators | 12,000 to 20,000 |
| Centrifugal separators, air conditioners, air blowers, woodworking equipment,passenger coach axle journals | 20,000 to 30,000 | |
| Large motors, mine hoists, locomotive axle journals, railway rolling stock traction motors | 40,000 to 60,000 | |
| Paper manufacturing equipment | 100,000 to 200,000 | |
| 24 hrs. operation (no failure allowed) | Water supply facilities, power stations, mine water discharge facilities | 100,000 to 200,000 |
4) Determination of required dynamic load rating
Obtain the required dynamic load rating by means of equation 3-4.
Calculation of required dynamic load rating
| C : | basic dynamic load rating | N |
| P : | dynamic equivalent load | N |
| for radial bearings | ||
| Pr : dynamic equivalent radial load | N | |
| for thrust bearings | ||
| Pa : dynamic equivalent axial load | N | |
| L10h : | required service life | h |
| n : | rotational speed | min-1 |
| p : | for ball bearings ……….. | p=3 |
| for roller bearings……….. | p=10/3 | |
When the axial load is not so large, the rating is provisionally estimated supposing that P equals Fr.
5) Selection of bearing
Select a bearing that meets the dynamic load rating requirement obtained by the above calculation, from those listed in the Bearing Specification Tables.
6) Calculation of bearing rating life
Obtain the dynamic equivalent load based on the radial and axial load factor of the selected bearing, and calculate the rating life of the bearing.
As for applications where loads on the bearing may vary, calculate the mean dynamic equivalent load by means of equation 3-5 , and estimate the rating life based on it.
Mean dynamic equivalent load (In case of staged fluctuation)

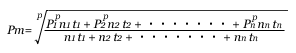
| Pm : | mean dynamic equivalent load | N |
| P1 : | dynamic equivalent load applied for t1 hours at speed n1 | N |
| P2 : | dynamic equivalent load applied for t2 hours at speed n2 | N |
| : | ||
| Pn : | dynamic equivalent load applied for tn hours at speed nn | N |
| p : | for ball bearings………p= 3 | |
| for roller bearings………p= 10/3 | ||
(Reference) Mean speed nm can be calculated using the following equation:
![]()
c. Basic static load rating and safety coefficient
1) Basic static load rating
A bearing may sustain a local deformation permanently when exposed to an excessively high static load or impact load. If the permanent deformation is beyond a specific extent, smooth rotation is imposed. The static load under which contact surface pressure working between the rolling element and raceway may generate the following sizes of contact stress is called the basic static load rating and is used as a reference to determine a high load.
Other ball bearings … 4200MPa
2) Static equivalent load
The static equivalent load is a virtually calculated load, under which contact stress of the same degree as generated under actual loading conditions would occur between the rolling elements and raceway in the section exposed to the maximum load.
It is calculated by means of the equivalent static load calculation equation, using the load factors listed in the tables.
(TO BE CONTINUED)
3) Safety coefficient
A safety coefficient is designated, based on empirical data, so as to ensure safety in relation to basic static load rating.
| where: | fs | : | safety coefficient | |
| C0 | : | basic static load rating | N | |
| P0 | : | static equivalent load | N |
| Operating conditions | fs(min.) | ||
| Ball bearings | Roller bearings | ||
| With bearing rotation | When high accuracy is required | 2 | 3 |
| Normal operation | 1 | 1.5 | |
| When impact load is applie | 1.5 | 3 | |
| Without bearing rotation (occasional oscillation) |
Normal operation | 0.5 | 1 |
| When impact load or uneven distribution load is applied | 1 | 2 | |
[Remark] For spherical thrust roller bearings ; fs≥4
Consult Koyo for further advice regarding safety factors appropriate to individual machines.

 Exclusive North American distributor for Gamet Bearing.
Exclusive North American distributor for Gamet Bearing.